Introduction to Rate of Change
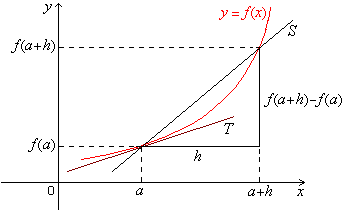
We have already learned how to find the derivative of composite functions, inverse trigonometric functions, implicit functions, exponential functions and logarithmic functions. Here, we learn how derivatives can be used to determine rate of change of quantities.
Rate of change of quantities
Related rates problems involve finding a rate at which a quantity changes by relating that quantity to other quantities whose rates of change are known. The rate of change is usually with respect to time. Problems on related rate methods often have nested functions, solving these problems requires application of the chain rule.
In simpler language, By ds / dt, we mean the rate of change of distance‘s’ with respect to time ‘t’. Whenever one quantity ‘y’ varies with another quantity x, satisfying some rule y = f(x), then dy / dx represents the rate of change of ‘y’ with respect to ‘x’ and [dy / dx] x=x0 represents the rate of change of ‘y’ with respect to ‘x’ at x = x0
We will learn the concept more with the help of following examples:
Example 1: Find the rate of change of the area of a circle with respect to its radius ‘r’ when r = 5cm
Solution: A = πr2
dA / dr = d / dr(πr2)
= 2πr
When r = 5.
dA / dr = 10π
Thus, area of the circle is changing at the rate of 10πcm2/cm
Example 2: The total revenue in Rupees received from the sale of ‘x’ units of a product is given by R(x) = 13x2 + 26x + 15. Find the marginal revenue when x = 7
Solution: Given R(x) = 13x2 + 26x + 15
dR / dx = 13 (2x) + 26 (1) +0
dR / dx = 26x + 26
When x = 7, dR / dx = 26 (7) + 26 = 208
Thus, marginal revenue when x = 7 is 208
Unsolved Problems on Rate of Change Using Derivatives:
1. An edge of a variable cube is increasing at the rate of 5 cm/second. How fast is the volume of the cube increasing when the edge is 7 cm long.
(Answer: 735 cm3 / s)
2. The radius of the circle is increasing uniformly at the rate of 4 cm per second. Find the rate at which the area of the circle is increasing when the radius is 8 cm.
(Answer: 64 π cm2 / s)
Now try it yourself! Should you still need any help, click here to schedule live online session with e Tutor!
About eAge Tutoring:
eAgeTutor.com is the premium online tutoring provider. Using materials developed by highly qualified educators and leading content developers, a team of top-notch software experts, and a group of passionate educators, eAgeTutor works to ensure the success and satisfaction of all of its students.
Contact us today to learn more about our tutoring programs and discuss how we can help make the dreams of the student in your life come true!
Reference Links:
- http://en.wikipedia.org/wiki/Derivative
- http://en.wikipedia.org/wiki/Function_composition
- http://en.wikipedia.org/wiki/Inverse_trigonometric_functions
- http://en.wikipedia.org/wiki/Implicit_and_explicit_functions
- http://en.wikipedia.org/wiki/Exponential_function
- http://en.wikipedia.org/wiki/Logarithm