
Introduction to the Carnot Cycle
What is the Carnot cycle?
The Carnot cycle is a particular thermodynamic cycle and is very important in the technology of engines. It is the most efficient cycle that currently exists and is abl
What is a Carnot engine?
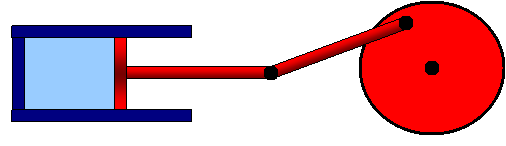
The most efficient heat engine cycle is the Carnot cycle, consisting of two isothermal processes and two adiabatic processes. The Carnot cycle can be thought of as the most efficient heat engine cycle allowed by physical laws. While the second law of thermodynamics states that not all the supplied heat in a heat engine can be used to do work, the Carnot efficiency sets the limiting value on the fraction of the heat that can be so used.
Description of the Carnot cycle
Stage 1: In the first stage, the piston moves downward while the engine absorbs heat from a source and gas begins to expand. Below, the portion of the graphic from point a to point b represents this behavior. Because the temperature of the gas does not change, this kind of expansion is called isothermic.
Stage 2: In the second stage, the heat source is removed; the piston continues to move downward and the gas continues to expand while cooling (lowering in temperature). This stage is presented by the graphic from point b to point c. This stage is called an adiabatic expansion (i.e., energy stays)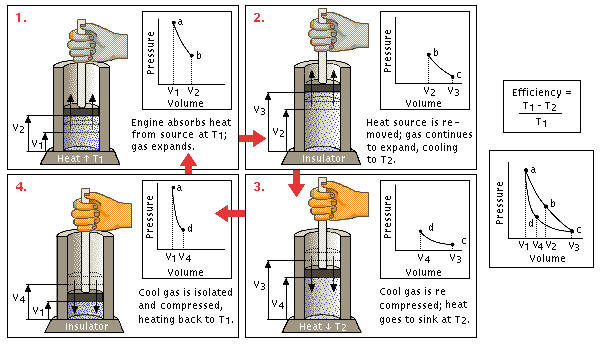
Stage 4: In the final stage, the piston continues to move upward and the cool gas is secluded and compressed. Its temperature rises to its original state. Point c to point d illustrates this behavior: a continuing increase in pressure and decrease in volume until they return to their initial position. Because energy stays, it's an adiabatic compression.
An ideal cycle would be the cycle that a perfectly efficient heat engine performs — that is, all the heat would be converted to mechanical work. Such an ideal engine cannot exist. Any heat engine must expend some fraction of its heat input as exhaust. The second law of thermodynamics places an upper limit on the efficiency of engines, and implies that that upper limit will be less than 100 percent. The limiting case is now known as a Carnot cycle. The efficiency of the Carnot cycle is important because it is the highest possible efficiency that any engine can reach, if the highest possible temperature the working substance of an engine can reach is TH, and the lowest possible temperature the working substance of the engine can reach is TC.
Carnot theorem:
All reversible engines operating between the same two thermal reservoirs at temperatures TH and TC have the same Carnot efficiency: 1-TC/TH. All irreversible engines operating between these two thermal reservoirs have efficiencies less than that of the Carnot engine running between these two reservoirs. All engines have heat intake at temperatures TH and below, and heat exhaust at temperatures TC and above, so that TH and TC are the highest and lowest temperatures involved, respectively.
When can the Carnot efficiency be 100%? Only when TH equals to infinity, or when TC equals to zero. But both are impossible to reach! Thus we again obtain the conclusion that no device can convert a given amount of heat completely into work, which would imply | Q C | = 0, and e = 100 %.
***
Want to know more about the Carnot cycle? Click here to schedule a live session with an eAge eTutor!
About eAge Tutoring:
eAgeTutor.com is the premium online tutoring provider. Using materials developed by highly qualified educators and leading content developers, a team of top-notch software experts, and a group of passionate educators, eAgeTutor works to ensure the success and satisfaction of all of its students.
Contact us today to learn more about our guaranteed results and discuss how we can help make the dreams of the student in your life come true!
