
Introduction to Focal Length
Focal length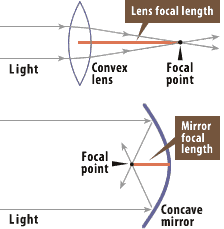

The distance along the principal axis of a mirror from the focus to the vertex is called the focal length of the mirror, and is denoted by f. The point that lies between the centre of the curvature and the vertex is called the focal point, or focus, of the mirror. The longer the focal length of a telescope, generally the more power it has, and the larger the image and the smaller the field of view. For example, a telescope with a focal length of 2000mm has twice the power and half the field of view of a 1000mm telescope.
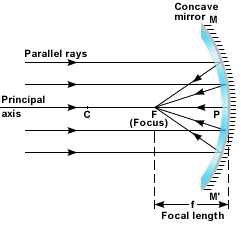
Focal length of a concave mirror

In a concave mirror, the rays appear to converge at a point F.
The distance PF is called the focal length and F is called the focal point.
The focus is in front of the mirror.
Focal length of a convex mirror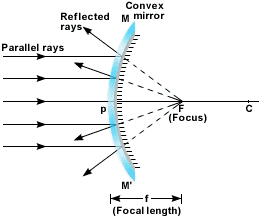

In the case of convex mirrors, reflected parallel rays appear to diverge from a point F.
This point is again called convex mirrors’ focal point and the distance PF is called the focal length.
The focus, in this case is behind the mirror.
Relation Between Focal Length and the Radius of Curvature
As shown in this figure, a paraxial ray is incident at point Q on a concave mirror. 
So, for Δ CFQ,
exterior∠ QFP = ∠ CQF + ∠ QCF = 2θ.
For a paraxial incident ray and small aperture,
CP’ » CP = R and FP’ » FP = f.
For a small aperture, 2θ is very small.
Therefore, from the figure: 2θ ≈ QP/FP = QP/f (1)
And θ = QP/CP = QP/R (2)
From equations (1 ) and ( 2 ), R = 2f → f = R / 2
Thus, the focal length of a concave mirror is half its radius of curvature.
***
Want to know more about the focal length of mirrors? Click here to schedule a live session with an eAge eTutor!
About eAge Tutoring:
eAgeTutor.com is the premier online tutoring provider. Using materials developed by highly qualified educators and leading content developers, a team of top-notch software experts, and a group of passionate educators, eAgeTutor works to ensure the success and satisfaction of all of its students.
Contact us today to learn more about our guaranteed results and discuss how we can help make the dreams of the student in your life come true!
