
Introduction
We have already learned that sine and cosine functions are defined for all real numbers. Also, we observe that for each real number x, 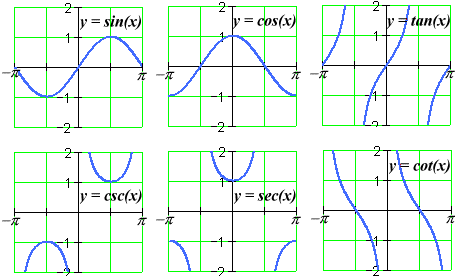
Thus, domain of y=sin(x) and y= cos(x) is the set of all real numbers and range is the interval [-1, 1]. Since cosec(x) = 1/sin(x), the domain of y= cosec(x) is the set {x: x Є R and x ≠ nπ, n Є Z} and the range is the set {y: y Є R, y≥1 or y≤-1}. Similarly, the domain of y=sec(x) is the set {x : x Є R and x≠(2n+1)π/2, n Є Z} and range is the set {y: y Є R, y≤-1 or y≥1}. The domain of y= tan(x) is the set {x: x Є R and x≠ (2n+1)π/2, n Є Z} and range is the set of all real numbers. The domain of y=cot(x) is the set {x: x Є R and x≠nπ, n Є Z} and the range is the set of all real numbers.
The values of sine, cosine and tangent can be seen in the table below
| Ф | 0˚ | π/6 | π/4 | π/3 | π/2 | π | 3π/2 | 2π |
| Sin(Ф) | 0 | 1/2 | 1/√2 | √3/2 | 1 | 0 | -1 | 0 |
| Cos(Ф) | 1 | √3/2 | 1/√2 | 1/2 | 0 | -1 | 0 | 1 |
| Tan(Ф) | 0 | 1/√3 | 1 | √3 | ∞ | 0 | ∞ | 0 |
The values of cosec(x), sec(x) and cot(x) are the reciprocal of the values of sin(x), cos(x) and tan(x) respectively.
First we can plot the points taking angles along x-axis and values against y-axis using the above given values. Below given is the sine curve and cosine curve.
The sine values are increasing to 1 and decreasing through the same values to -1, then returning to 0. When we connect all these values using a smooth curve we get a periodic curve of y=sin(x)
Graphs of sine and cosine functions
The sine curve with the equation y=sin(x) is the simplest trigonometric graphs. The curve rises and falls by one unit from its axis of oscillation- in this case after going through 360˚, again we come across the same y values. The same pattern can be seen for cosine graph also.
Each sine and cosine graph has two basic characteristics
1. The distance the curve rises above and falls below, its axis of oscillation is called amplitude and it is given by
amp = max – min
2
2. The length of one cycle is called period of the graph and this can be calculated by measuring the distance between maximum or minimum points or doubling horizontal distance between a minimum and the next maximum point.
Here the amplitude is 1 and period is 360˚ or 2π radians.
Graph of y = Sin (x)
Graph of y = Cos (x)
Modeling Periodic Phenomena
We get the trigonometric models of y = a sink (θ + b) + d and y = a cosk (θ + b) + d by first graphing periodic sinusoidal data.
We can model and solve many real-life problems using y = a sink(θ + b)+d or y = acosk(θ + b) + d.
The values of a, k, b and d are found by determining the transformations that must be applied to y= sin(θ) or y= cos(θ), respectively, to obtain the graph of the data.
Example: Determine the function that is simplest model of the following data
| Independent variable | -90˚ | -45˚ | 0˚ | 45˚ | 90˚ | 125˚ | 180˚ |
| Dependent Variable | 8 | 6 | 4 | 6 | 8 | 6 | 4 |
Solution: The curve will be either sine or cosine function
Case I: Sine Model
For sine model, y = asink(θ + b) + d
The maximum value is 8 and minimum value is 4
The equation of axis is y = 8 + 4 = 6
2
Then d, the vertical translation is 6
The amplitude a = 8 – 4 = 2
2
The period is 180˚. There are two complete cycles for each complete cycles of y = sinθ. The horizontal compression is a factor of ½ so k=2, b= -45˚
Now substitute these values to the equation y = asink(θ+b)+d to get
y = 2 sin2(θ-45˚)+6
Case II: Cosine Model
Here also k=2, d=6, a=-2 and b=0 so that the equation y=acosk(θ+b)+d becomes
y = -2 Cos 2(θ+0)+6
y = -2cos2θ+6
Now try it yourself! Should you still need any help, click here to schedule live online session with e Tutor!
About eAge Tutoring:
eAgeTutor.com is the premium online tutoring provider. Using materials developed by highly qualified educators and leading content developers, a team of top-notch software experts, and a group of passionate educators, eAgeTutor works to ensure the success and satisfaction of all of its students.
Contact us today to learn more about our tutoring programs and discuss how we can help make the dreams of the student in your life come true!
