TRIGONOMETRIC FUNCTIONS OF SUM AND DIFFERENCE OF TWO ANGLES

Created: Saturday, 17 September 2011 08:57 | Published: Saturday, 17 September 2011 08:57 | Written by Super User | Print | Email

Trigonometric Functions of Sum and Differences Introduction

So far we have learnt basic trigonometric identities, in this section we will learn about trigonometric functions of the sum and difference of two angles using some basic results, following are the results which we must know before starting this topic:

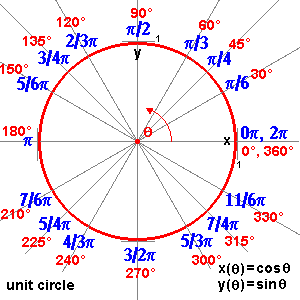
1. Sin (-x) = - Sin x
2. Cos (-x) = Cos x
3. Sin (Π2 – x) = Cos x
4. Cos (Π2 – x) = Sin x
5. Sin (Π2 + x) = Cos x
6. Cos (Π2 + x) = - Sin x
7. Cos (Π – x) = - Cos x
8. Sin (Π – x) = Sin x
9. Cos (Π + x) = - Cos x
10. Sin (Π + x) = - Sin x
11. Cos (2Π – x) = Cos x
12. Sin (2Π - x) = - Sin x

Basic Proof

Consider the unit circle with centre at the origin.

Let x be the angle P₄OP₁ and y be the angle P₁OP₂. Then (x + y) is the angle P₄OP₂. Also let (– y) be the angle P₄OP₃.

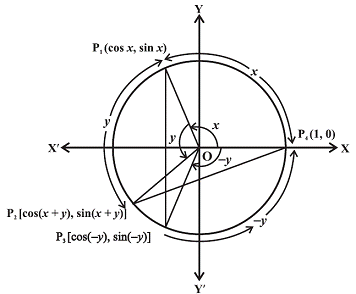
Therefore, P₁, P₂, P₃ and P₄ will have the coordinates:

P₁ (Cos x, Sin x),
P₂ [Cos (x + y), Sin (x + y)],
P₃ [Cos (– y), Sin (– y)] and
P₄ (1, 0)

Consider the triangles P₁OP₃ and P₂OP₄. They are congruent

Therefore, P₁P₃ and P₂P₄ are equal. By using distance formula, we get
P₁P₃²
2 = [Cos x – Cos (– y)]² + [Sin x – Sin(–y]²
= (Cos x – Cos y)² + (Sin x + Sin y)²
= Cos²x + Cos²y – 2 Cos x Cos y + Sin²x + Sin²y + 2Sin x Sin y
= 2 – 2 (Cos x Cos y – Sin x Sin y)

Also, P₂P₄²
2 = [1 – Cos (x + y)]² + [0 – Sin (x + y)]²
= 1 – 2Cos (x + y) + Cos²(x + y) + Sin²(x + y)
= 2 – 2 Cos (x + y)

Since P₁P₃ = P₂P₄, we have P₁P₃² = P₂P₄²

Therefore, 2 –2 (Cos x Cos y – Sin x Sin y) = 2 – 2 Cos (x + y).

So, from the above discussion we get:
Cos (x + y) = Cos x Cos y – Sin x Sin y – (I)

Cosine: Sum and Difference of Angles

Sum of Angles: Cos (x + y)

From (I) we get:
Cos (x + y) = Cos x Cos y – Sin x Sin y

Difference of Angles: Cos (x – y)

Replacing y by (-y) in (I), we get:
Cos (x + y) = Cos x Cos y – Sin x Sin y
Cos (x + (-y)) = Cos x Cos (-y) – Sin x Sin (-y)
Cos (x – y) = Cos x Cos y + Sin x Sin y (From 1 and 2)

Sine: Sum and Difference of Angles

We will use Cos (Π2 – x) = Sin x to find out sum and difference of angles

Sum of Angles: Sin (x + y)

Sin (x + y) = Cos (Π2 – (x + y))
Sin (x + y) = Cos ((Π2 – x) – y)
= Cos (Π2 – x) Cos y + Sin (Π2 – x) Sin y (Using (I))
= Sin x Cos y + Cos x Sin y

Difference of Angles: Sin (x – y)

Replacing y by (-y) in sum of angles
Sin (x + (-y)) = Cos (Π2 – (x + (-y)))
Sin (x - y) = Cos ((Π2 – x) + y)
= Cos (Π2 – x) Cos y - Sin (Π2 – x) Sin y (Using (I))
= Sin x Cos y - Cos x Sin y

Tangent: Sum and Difference of Angles

We consider that x, y are not an odd multiple of Π2

Sum of Angles: Tan (x + y)

Tan (x + y) = Sin (x+y)Cos (x+y)
Tan (x + y) = Sin x Cos y+Cos x Siny Cos x Cos y-Sin x Sin y

Dividing numerator and denominator by Cos x Cos y, we have:
Tan (x + y) = Tan x +Tan y1-Tan x Tan y

Difference of Angles: Tan (x – y)

Replacing y by (-y) in sum of angles
Tan (x – y) = Tan x- Tan y1+Tan x Tan y

Now try it yourself! Should you still need any help, click here to schedule live online session with e Tutor!

About eAge Tutoring:

eAgeTutor.com is the premium online tutoring provider. Using materials developed by highly qualified educators and leading content developers, a team of top-notch software experts, and a group of passionate educators, eAgeTutor works to ensure the success and satisfaction of all of its students.

Contact us today to learn more about our tutoring programs and discuss how we can help make the dreams of the student in your life come true!

Reference Links:

Category: ROOT

Articles