
Introduction to Trig Ratios
In the adjoining figure, we have Δ ABC right angled at C.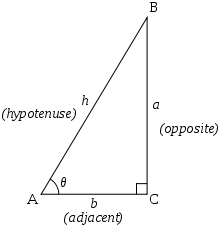
• Sine θ
• Cosine θ
• Cosecant θ
• Secant θ
• Cotangent θ
Let the Hypotenuse in Δ ABC = h
Adjacent in Δ ABC = b
Opposite in Δ ABC = a
Now, we define the above mentioned trigonometric ratios:
• Sine θ or Sin θ = Opposite / Hypotenuse = a / h
• Cosine θ or Cos θ = Adjacent / Hypotenuse = b / h
• Tangent θ or Tan θ = Opposite / Adjacent = a / b
• Cosecant θ or Cosec θ = Hypotenuse / Opposite = h / a
• Secant θ or Sec θ = Hypotenuse / Adjacent = h / b
• Cotangent θ or Cot θ = Adjacent / Opposite = b / a
From the above discussion, it is clear that the last three trigonometric ratios are opposite of the first three trigonometric ratios respectively.
That is,
• Cosecant θ or Cosec θ = 1 / Sine θ
• Secant θ or Sec θ = 1 / Cosine θ
• Cotangent θ or Cot θ = 1 / Tangent θ
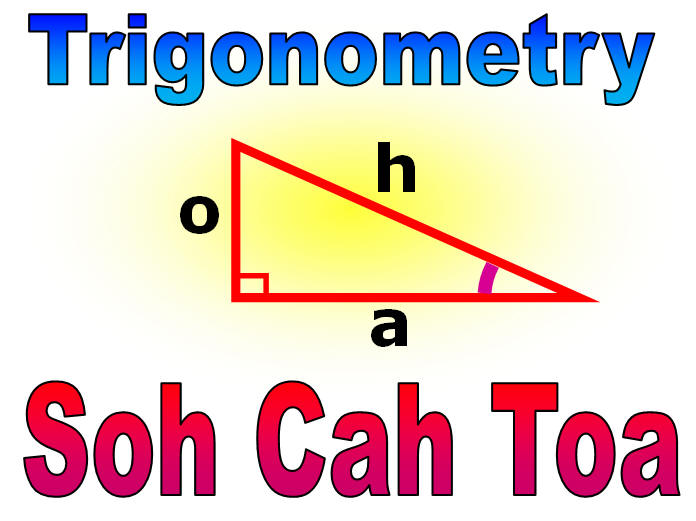

There is one short method for remembering all six trigonometric ratios.
SOH
‘S’ stands for Sine
‘O’ stands for Opposite
‘H’ stands for Hypotenuse
Sine = Opposite / Hypotenuse
CAH
‘C’ stands for Cosine
‘A’ stands for Adjacent
‘H’ stands for Hypotenuse
Cosine = Adjacent / Hypotenuse
TOA
‘T’ stands for Tangent
‘O’ stands for Opposite
‘A’ stands for Adjacent
Tangent = Opposite / Adjacent
As discussed above, that Cosecant, Secant, and Cotangent are opposites of Sine, Cosine and Tangent respectively.
Let’s solve few problems based on the above discussion:
In Δ ABC, right angled at A, if AB = 12, AC = 5 and BC = 13, find all the six trigonometric ratios of angle B.
With reference to above Δ ABC we have,
Opposite = AC = 5
Adjacent = AB = 12
Hypotenuse = BC = 13
Using the definitions of trigonometric ratios, we have
Sine B = Opposite / Hypotenuse = AC / BC = 5 / 13
Cosine B = Adjacent / Hypotenuse = AB / BC = 12 / 13
Tangent B = Opposite / Adjacent = AC / AB = 5 / 12
Cosecant B = Hypotenuse / Opposite = BC / AC = 13 / 5
Secant B = Hypotenuse / Adjacent = BC / AB = 13 / 12
Cotangent B = Adjacent / Opposite = AB / AC = 12 / 5
Now try it yourself! Should you still need any help, click here to schedule live online session with e Tutor!
About eAge Tutoring:
eAgeTutor.com is the premium online tutoring provider. Using materials developed by highly qualified educators and leading content developers, a team of top-notch software experts, and a group of passionate educators, eAgeTutor works to ensure the success and satisfaction of all of its students.
Contact us today to learn more about our tutoring programs and discuss how we can help make the dreams of the student in your life come true!
