Introduction

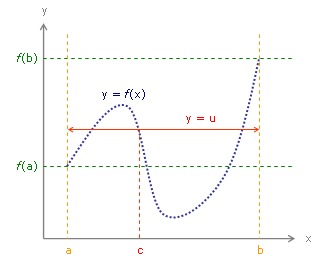
Suppose f(x) be a function continuous in [a, b] and let M be a number between f(a) and f(b).  Then there exists a number c Є [a, b] such that     f(c) = M.
Intermediate Value Theorem says that a continuous function will take on all values between f(a) and f(b).  The graph shown below illustrates Intermediate Value Theorem.
                        

Explanation

From the graph seen above, if we pick any value M in between f(a) and f(b) and draw a line straight to the graph, it will intersect the graph in at least one point.  In other words somewhere between ‘a’ and ‘b’ the function will take the value M.  The figure also shows that the function can take more than one value.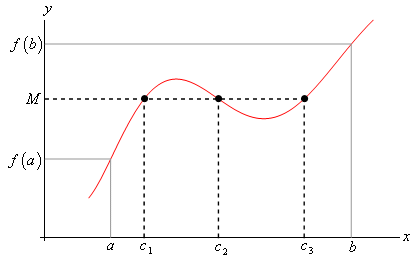

It is also important to note that the Intermediate Value Theorem only says that the function will take the value of M somewhere between ‘a’ and ‘b’ and does not say what will be the value.

Intermediate Value Theorem is mostly used to prove the existence of roots of equations.

Problems related to Intermediate Value Theorem

1.  Show that the function f(x) = lnx – 1 has a solution between 2 and 3
Solution: We can see that f(2) = ln 2 – 1
                                             ≈ -0.307
                              Also f(3)  =  ln 3 – 1
                                            ≈ 0.099
Since the first number is negative and second number is positive and f(x) is continuous in [2, 3], by Intermediate Value Theorem, f(x) must have a solution between 2 and 3.
2. Show that the function f(x) = x⁵ + 2x³ + x – 5 has only one real solution.
Solution: First we use Intermediate Value Theorem to show that there is at least one solution.  Since f(x) is continuous and f(1) = -1 and f(2) = 45 which means that the solution is somewhere between 1 and 2, f(x)=0.  Hence we have shown that f(x) has at least one solution.
Now we have to show that it is the only solution.  For that we have to show that there is no second solution for f(x).  We can do this by taking the derivative of f(x).
f’(x) = 5x⁴ + 6x² + 1
Since x⁴ and x² are positive terms clearly f’(x)>0, which means that f(x) is strictly increasing function.  A strictly increasing function will have only one x-intercept (solution), and thus f(x) has only one real solution.

3. Use the Intermediate Value Theorem to show that the function 2 – e^{x^2} = sin(x) has at least one solution on the interval [0, 1]
Solution:  Let f(x) = 2 – e^{x^2} – sin(x)
                f(0) = 2 – e⁰ – sin(0)
                       = 2 – 1 – 0
                       = 1 > 0
                 f(1) = 2 – e¹ – sin(1)
                                   ≈ -1.55975 < 0
F(x) is continuous in [0, 1] since trigonometric function, exponential function, sum and difference of continuous functions are continuous.
The intermediate value theorem tells us that since f is continuous on     [0, 1] and N=0 between f(0) and f(1), then there exists a c in (0, 1) such that f(c ) = N =0

Now try it yourself!  Should you still need any help, click here to schedule live online session with e Tutor!

About eAge Tutoring:

eAgeTutor.com is the premium online tutoring provider.  Using materials developed by highly qualified educators and leading content developers, a team of top-notch software experts, and a group of passionate educators, eAgeTutor works to ensure the success and satisfaction of all of its students.  

Contact us today to learn more about our tutoring programs and discuss how we can help make the dreams of the student in your life come true!

Reference Links:

• http://en.wikipedia.org/wiki/Intermediate_value_theorem
• http://en.wikipedia.org/wiki/Trigonometric_functions
• http://en.wikipedia.org/wiki/Continuous_function
• http://en.wikipedia.org/wiki/Rational_function